ARITHMETIC SEQUENCE
Sequence is a set of things (usually numbers) that in order.
Arithmetic Sequence the difference between one term and the next is a constant.
in other words, we just add the same value each time ... infinitely.
If the first term
of the sequence is a then the arithmetic progression is
a, a + d, a + 2d, a + 3d, . . .
where the n-th term is
a + (n − 1)d.
The sum of an arithmetic series Sometimes we want to add the terms of a sequence.
Example 1:
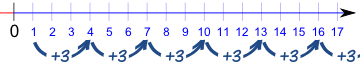
| 1, 4, 7, 10, 13, 16, 19, 22, 25, ... |
This sequence has a difference of 3 between each number.
The pattern is continued by adding 3 to the last number each time, like this:
The pattern is continued by adding 3 to the last number each time, like this:
In General we could write an Arithmetic Sequence like this:
{a, a+d, a+2d, a+3d, ...}
Where:
- a is the first term, and
- d is the difference between the terms (called the "common difference")
| 1, 4, 7, 10, 13, 16, 19, 22, 25, ... |
Has:
- a = 1 (the first term)
- d = 3 (the "common difference" between terms)
And we get:
{a, a+d, a+2d, a+3d, ... }
= {1, 1+3, 1+2×3, 1+3×3, ... }
= {1, 4, 7, 10, ... }
Example 2:
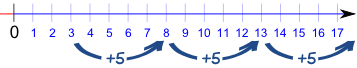
| 3, 8, 13, 18, 23, 28, 33, 38, ... |
This sequence has a difference of 5 between each number.
The pattern is continued by adding 5 to the last number each time, like this:
The pattern is continued by adding 5 to the last number each time, like this:
We can write an Arithmetic Sequence as a rule:
xn = a + d(n-1)
(We use "n-1" because d is not used in the 1st term).
Write the Rule, and calculate the 4th term for
| 3, 8, 13, 18, 23, 28, 33, 38, ... |
This sequence has a difference of 5 between each number.
The values of a and d are:
- a = 3 (the first term)
- d = 5 (the "common difference")
The Rule can be calculated:
xn = a + d(n-1)
= 3 + 5(n-1)
= 3 + 5n - 5
= 5n - 2
So, the 4th term is:
x4 = 5×4 - 2 = 18
Examples of Arithmetic Progression
Find the sum of the first 50 terms of the sequence
1, 3, 5, 7, 9, . . . .
Solution
This is an arithmetic progression, and we can write down
a = 1 , d = 2 , n = 50 .
We now use the formula,
so that
Sn =
1/2
n(2a + (n − 1)d)
S50 =
1/2 × 50 × (2 × 1 + (50 − 1) × 2)
= 25 × (2 + 49 × 2)
= 25 × (2 + 98)
= 2500 .
Find the n-terms of first sequence
1, 3, 5, 7, 9, . . .
1,
1 + 2,
1 + 2 × 2,
1 + 3 × 2,
1 + 4 × 2,
. . . ,
and
this can be written as
a,
a + d,
a + 2d,
a + 3d,
a + 4d,
. . .
where
a = 1 is the first term,
and
d = 2 is the common difference.
If we wanted to write down
the n-th term,
we would have
a + (n − 1)d
References:




This awesome, i totally understand it now. Thanks~
ReplyDeleteThis methods are what ive been searching for, keep the good work!
ReplyDeleteThanks, I'm able to understand this topic with the help of your notes.
ReplyDelete